As we all know, through play, kids learn different things without even realizing it.

Playing with a spirograph, experimenting, and trying all kinds of combinations, kids will develop mathematical and scientific intuition they can draw and realize the patterns. With the proper questions, they can experiment, hypothesize, test, and generalize even reach conclusions.

  

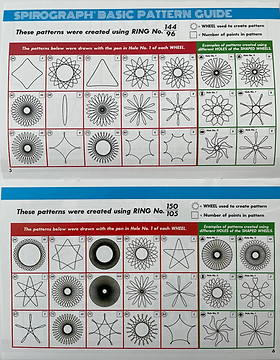
A Spirograph is a geometric drawing device that produces various mathematical curves such as hypotrochoids and epitrochoids. 

  

The well-known toy version was developed by British engineer Denys Fisher and was first sold in 1965. Visit the original toy’s website.

There is also a very successful online version here.

​

The patterns that are created depend on three variables: 

the radius of the fixed disc or wheel (the number of teeth)

the radius of the revolving disc (the number of teeth)

the location of the point on the moving disc. 

​

By changing any one of these variables, you can get tons of incredible and beautiful patterns. Please check Wolfram's collection of plane curves to identify them.

  

A point on a wheel rolling inside a circle traces out a hypocycloid.

A point on a wheel rolling on a flat surface traces out a curve called a cycloid.

A point on a wheel rolling outside another wheel traces out an epicycloid.

 

A spirograph can also be used to study;

LCM

Modular  arithmetic

The fundamental theorem of mathematics.

Click  here for the SPIROGRAPH TASK about LCM and Modular Arithmetic (for the middle school level)